Pigeonhole Collection

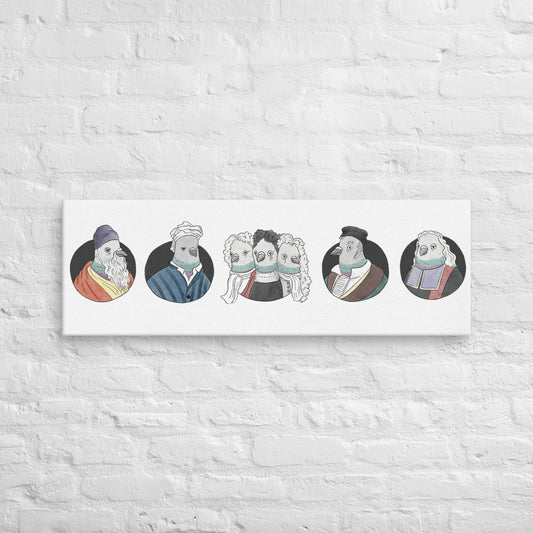
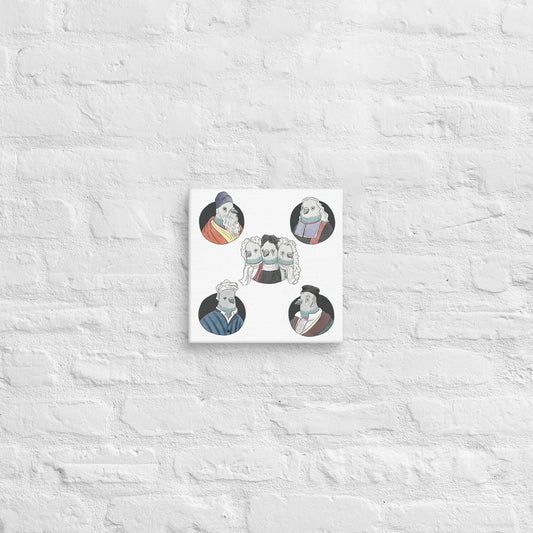
The Pigeonhole Principle states: if there are more pigeons than there are holes, then at least one hole has at least two pigeons. This fact is obvious yet incredibly useful throughout combinatorial mathematics. Even proving there are two people in the United States with the exact same number of hairs on their heads is an easy exercise with the Pigeonhole Principle. The design in this collection shows the principle by packing seven famous mathemapigeons into only five pigeonholes. The mathemapigeons are Euclid, Fermat, Euler, Gauss, and the Bernoullis (Johann, Jacob, and Daniel).